-
A truss consists of horizontal members (AC, CD, DB and EF) and vertical members (CE and DF) having length l each. The-members AE, DE and BF are inclined at 45° to the horizontal For the uniformly distributed load p per unit length on the members EF of the truss shown in figure given below, the force in the member CD is
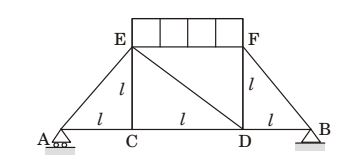
-
-
pl 2
- pl
- 0
-
2pl 3
-
Correct Option: A
Total load on EF member = pl
where l = length of EF
For horizontal equilibrium RA + RB = Pl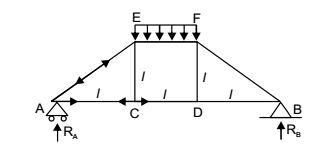
Taking moment about A, we have
| pl × | = RB × 3l | |
| 2 |
| ⇒ RB = | ||
| 2 |
Considering a point A.
For horizontal equilibrium FAC + FAE cos 45° = 0
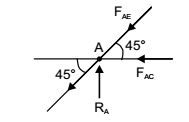
For vertical equilibrium RA – FAE sin 45° = 0
| ⇒ FAE = | = | = | |||
| sin 45° | 2 × (1 / √2) | √2 |
| ∴ FAC = -FAE cos45° = | . | = | |||
| √2 | √2 | 2 |
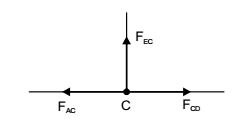
–ve sign shows that the force on member AC is opposite of assumed. Now considering a point C For horizontal equilibrium
| FAC = FCD = | ||
| 2 |

